corrections of the first lecture after first review
This commit is contained in:
parent
f257b26d0a
commit
60d7f7d850
Binary file not shown.
@ -36,7 +36,7 @@
|
||||
}%
|
||||
\providecommand\rotatebox[2]{#2}%
|
||||
\ifx\svgwidth\undefined%
|
||||
\setlength{\unitlength}{364.49980969bp}%
|
||||
\setlength{\unitlength}{280.39412611bp}%
|
||||
\ifx\svgscale\undefined%
|
||||
\relax%
|
||||
\else%
|
||||
@ -48,12 +48,12 @@
|
||||
\global\let\svgwidth\undefined%
|
||||
\global\let\svgscale\undefined%
|
||||
\makeatother%
|
||||
\begin{picture}(1,0.42290942)%
|
||||
\put(0.29250579,2.25393507){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.26372184\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.29250579,2.25393507){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.26372184\unitlength}\raggedright \end{minipage}}}%
|
||||
\begin{picture}(1,0.64151204)%
|
||||
\put(0.294701,3.02176327){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.34282656\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.294701,3.02176327){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.34282656\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0,0){\includegraphics[width=\unitlength,page=1]{linking_hopf.pdf}}%
|
||||
\put(0.86572181,0.24480623){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.48025377\unitlength}\raggedright $\Lk(\alpha, \beta) = -1$\\ \end{minipage}}}%
|
||||
\put(-0.0000008,0.37342144){\color[rgb]{1,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.21983917\unitlength}\raggedright $\alpha$\\ \end{minipage}}}%
|
||||
\put(0.73747224,0.40373095){\color[rgb]{0,0,1}\makebox(0,0)[lt]{\begin{minipage}{0.21983917\unitlength}\raggedright $\beta$\\ \end{minipage}}}%
|
||||
\put(0.40133526,0.02351275){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.62430836\unitlength}\raggedright $\Lk(\alpha, \beta) = -1$\\ \end{minipage}}}%
|
||||
\put(-0.00000051,0.59179048){\color[rgb]{1,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.14230468\unitlength}\raggedright $\alpha$\\ \end{minipage}}}%
|
||||
\put(0.8318937,0.49629103){\color[rgb]{0,0,1}\makebox(0,0)[lt]{\begin{minipage}{0.16825112\unitlength}\raggedright $\beta$\\ \end{minipage}}}%
|
||||
\end{picture}%
|
||||
\endgroup%
|
||||
|
||||
@ -10,9 +10,9 @@
|
||||
xmlns="http://www.w3.org/2000/svg"
|
||||
xmlns:sodipodi="http://sodipodi.sourceforge.net/DTD/sodipodi-0.dtd"
|
||||
xmlns:inkscape="http://www.inkscape.org/namespaces/inkscape"
|
||||
width="128.58743mm"
|
||||
height="54.380836mm"
|
||||
viewBox="0 0 455.62479 192.68801"
|
||||
width="98.916817mm"
|
||||
height="63.456329mm"
|
||||
viewBox="0 0 350.49268 224.84528"
|
||||
id="svg2"
|
||||
version="1.1"
|
||||
inkscape:version="0.92.2 5c3e80d, 2017-08-06"
|
||||
@ -213,10 +213,10 @@
|
||||
is_visible="true" />
|
||||
<inkscape:perspective
|
||||
sodipodi:type="inkscape:persp3d"
|
||||
inkscape:vp_x="-511.16441 : -343.29587 : 1"
|
||||
inkscape:vp_y="-295.66613 : 82.942191 : 0"
|
||||
inkscape:vp_z="2308.1636 : 744.37608 : 1"
|
||||
inkscape:persp3d-origin="964.63207 : 181.98816 : 1"
|
||||
inkscape:vp_x="-511.16443 : -343.29589 : 1"
|
||||
inkscape:vp_y="-295.66615 : 82.942197 : 0"
|
||||
inkscape:vp_z="2308.1637 : 744.37613 : 1"
|
||||
inkscape:persp3d-origin="964.63211 : 181.98817 : 1"
|
||||
id="perspective5719" />
|
||||
<linearGradient
|
||||
id="linearGradient4984"
|
||||
@ -1805,9 +1805,9 @@
|
||||
borderopacity="1.0"
|
||||
inkscape:pageopacity="0.0"
|
||||
inkscape:pageshadow="2"
|
||||
inkscape:zoom="0.62698066"
|
||||
inkscape:cx="389.39003"
|
||||
inkscape:cy="-109.45396"
|
||||
inkscape:zoom="1.7510297"
|
||||
inkscape:cx="211.01871"
|
||||
inkscape:cy="137.06801"
|
||||
inkscape:document-units="px"
|
||||
inkscape:current-layer="layer1"
|
||||
showgrid="false"
|
||||
@ -1826,8 +1826,8 @@
|
||||
<inkscape:grid
|
||||
type="xygrid"
|
||||
id="grid66701"
|
||||
originx="-401.97735"
|
||||
originy="83.160629" />
|
||||
originx="-431.95973"
|
||||
originy="115.31789" />
|
||||
</sodipodi:namedview>
|
||||
<metadata
|
||||
id="metadata7">
|
||||
@ -1845,7 +1845,7 @@
|
||||
inkscape:label="Layer 1"
|
||||
inkscape:groupmode="layer"
|
||||
id="layer1"
|
||||
transform="translate(-401.97736,-942.83463)">
|
||||
transform="translate(-431.9597,-942.83463)">
|
||||
<flowRoot
|
||||
xml:space="preserve"
|
||||
id="flowRoot5468"
|
||||
@ -1888,7 +1888,7 @@
|
||||
xml:space="preserve"
|
||||
id="flowRoot11265"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;font-size:11.99991798px;line-height:0.01%;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';font-variant-ligatures:normal;font-variant-caps:normal;font-variant-numeric:normal;font-feature-settings:normal;text-align:start;letter-spacing:0px;word-spacing:0px;writing-mode:lr-tb;text-anchor:start;fill:#000000;fill-opacity:1;stroke:none;stroke-width:1px;stroke-linecap:butt;stroke-linejoin:miter;stroke-opacity:1"
|
||||
transform="matrix(0.41584592,0,0,1.4680523,568.26312,653.02114)"><flowRegion
|
||||
transform="matrix(0.41584592,0,0,1.4680523,344.46623,788.47715)"><flowRegion
|
||||
id="flowRegion11267"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;font-size:11.99991798px;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';font-variant-ligatures:normal;font-variant-caps:normal;font-variant-numeric:normal;font-feature-settings:normal;text-align:start;writing-mode:lr-tb;text-anchor:start"><rect
|
||||
id="rect11269"
|
||||
@ -1899,7 +1899,7 @@
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;font-size:11.99991798px;line-height:125%;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';font-variant-ligatures:normal;font-variant-caps:normal;font-variant-numeric:normal;font-feature-settings:normal;text-align:start;writing-mode:lr-tb;text-anchor:start" /></flowRegion><flowPara
|
||||
id="flowPara4663">$\Lk(\alpha, \beta) = -1$</flowPara><flowPara
|
||||
id="flowPara4665"> </flowPara></flowRoot> <flowRoot
|
||||
transform="matrix(0.42148328,0,0,0.28985975,170.72545,892.138)"
|
||||
transform="matrix(0.20987759,0,0,0.24324024,316.80782,898.79737)"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;line-height:0.01%;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';text-align:start;letter-spacing:0px;word-spacing:0px;writing-mode:lr-tb;text-anchor:start;fill:#ff0000;fill-opacity:1;stroke:none;stroke-width:1px;stroke-linecap:butt;stroke-linejoin:miter;stroke-opacity:1"
|
||||
id="flowRoot11275"
|
||||
xml:space="preserve"><flowRegion
|
||||
@ -1917,7 +1917,7 @@
|
||||
xml:space="preserve"
|
||||
id="flowRoot11289"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;line-height:0.01%;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';text-align:start;letter-spacing:0px;word-spacing:0px;writing-mode:lr-tb;text-anchor:start;fill:#0000ff;fill-opacity:1;stroke:none;stroke-width:1px;stroke-linecap:butt;stroke-linejoin:miter;stroke-opacity:1"
|
||||
transform="matrix(0.42148328,0,0,0.28985975,506.73644,878.32824)"><flowRegion
|
||||
transform="matrix(0.24814462,0,0,0.20994058,587.38502,940.68371)"><flowRegion
|
||||
id="flowRegion11291"><rect
|
||||
id="rect11293"
|
||||
width="237.64688"
|
||||
|
||||
|
Before 
(image error) Size: 69 KiB After 
(image error) Size: 69 KiB 

|
Binary file not shown.
@ -36,7 +36,7 @@
|
||||
}%
|
||||
\providecommand\rotatebox[2]{#2}%
|
||||
\ifx\svgwidth\undefined%
|
||||
\setlength{\unitlength}{318.45018282bp}%
|
||||
\setlength{\unitlength}{260.80271323bp}%
|
||||
\ifx\svgscale\undefined%
|
||||
\relax%
|
||||
\else%
|
||||
@ -48,12 +48,12 @@
|
||||
\global\let\svgwidth\undefined%
|
||||
\global\let\svgscale\undefined%
|
||||
\makeatother%
|
||||
\begin{picture}(1,0.48333952)%
|
||||
\put(0.36244031,2.57302301){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.30185744\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.36244031,2.57302301){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.30185744\unitlength}\raggedright \end{minipage}}}%
|
||||
\begin{picture}(1,0.69671239)%
|
||||
\put(0.47250725,3.24829661){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.36857959\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.47250725,3.24829661){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.36857959\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0,0){\includegraphics[width=\unitlength,page=1]{linking_torus_6_2.pdf}}%
|
||||
\put(0.80794467,0.26923855){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.66035143\unitlength}\raggedright \shortstack{$\Lk(\alpha, \beta) = 3$}\end{minipage}}}%
|
||||
\put(0.02836708,0.33088267){\color[rgb]{1,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.23861542\unitlength}\raggedright $\alpha$\\ \end{minipage}}}%
|
||||
\put(0.57683835,0.12154638){\color[rgb]{0,0,1}\makebox(0,0)[lt]{\begin{minipage}{0.23861542\unitlength}\raggedright $\beta$\\ \end{minipage}}}%
|
||||
\put(0.35502959,0.02062157){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.80631459\unitlength}\raggedright \shortstack{$\Lk(\alpha, \beta) = 3$}\end{minipage}}}%
|
||||
\put(-0.00000047,0.6338306){\color[rgb]{1,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.1344035\unitlength}\raggedright $\alpha$\\ \end{minipage}}}%
|
||||
\put(0.73429555,0.25494899){\color[rgb]{0,0,1}\makebox(0,0)[lt]{\begin{minipage}{0.29135864\unitlength}\raggedright $\beta$\\ \end{minipage}}}%
|
||||
\end{picture}%
|
||||
\endgroup%
|
||||
|
||||
@ -10,9 +10,9 @@
|
||||
xmlns="http://www.w3.org/2000/svg"
|
||||
xmlns:sodipodi="http://sodipodi.sourceforge.net/DTD/sodipodi-0.dtd"
|
||||
xmlns:inkscape="http://www.inkscape.org/namespaces/inkscape"
|
||||
width="112.34215mm"
|
||||
height="54.2994mm"
|
||||
viewBox="0 0 398.06274 192.39946"
|
||||
width="92.005402mm"
|
||||
height="64.101303mm"
|
||||
viewBox="0 0 326.0034 227.13061"
|
||||
id="svg2"
|
||||
version="1.1"
|
||||
inkscape:version="0.92.2 5c3e80d, 2017-08-06"
|
||||
@ -258,10 +258,10 @@
|
||||
is_visible="true" />
|
||||
<inkscape:perspective
|
||||
sodipodi:type="inkscape:persp3d"
|
||||
inkscape:vp_x="-511.16441 : -343.29587 : 1"
|
||||
inkscape:vp_y="-295.66612 : 82.942192 : 0"
|
||||
inkscape:vp_z="2308.1636 : 744.37608 : 1"
|
||||
inkscape:persp3d-origin="964.63204 : 181.98817 : 1"
|
||||
inkscape:vp_x="-511.16444 : -343.29585 : 1"
|
||||
inkscape:vp_y="-295.66613 : 82.942188 : 0"
|
||||
inkscape:vp_z="2308.1637 : 744.37604 : 1"
|
||||
inkscape:persp3d-origin="964.63209 : 181.98816 : 1"
|
||||
id="perspective5719" />
|
||||
<linearGradient
|
||||
id="linearGradient4984"
|
||||
@ -1835,9 +1835,9 @@
|
||||
borderopacity="1.0"
|
||||
inkscape:pageopacity="0.0"
|
||||
inkscape:pageshadow="2"
|
||||
inkscape:zoom="0.14715637"
|
||||
inkscape:cx="250.88609"
|
||||
inkscape:cy="270.29308"
|
||||
inkscape:zoom="1.4172104"
|
||||
inkscape:cx="182.80068"
|
||||
inkscape:cy="139.6596"
|
||||
inkscape:document-units="px"
|
||||
inkscape:current-layer="layer1"
|
||||
showgrid="false"
|
||||
@ -1858,8 +1858,8 @@
|
||||
<inkscape:grid
|
||||
type="xygrid"
|
||||
id="grid66701"
|
||||
originx="-390.9762"
|
||||
originy="80.436871" />
|
||||
originx="-381.21121"
|
||||
originy="115.16802" />
|
||||
</sodipodi:namedview>
|
||||
<metadata
|
||||
id="metadata7">
|
||||
@ -1877,7 +1877,7 @@
|
||||
inkscape:label="Layer 1"
|
||||
inkscape:groupmode="layer"
|
||||
id="layer1"
|
||||
transform="translate(-390.97626,-940.39913)">
|
||||
transform="translate(-381.21127,-940.39913)">
|
||||
<flowRoot
|
||||
xml:space="preserve"
|
||||
id="flowRoot5468"
|
||||
@ -1922,7 +1922,7 @@
|
||||
xml:space="preserve"
|
||||
id="flowRoot11265"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;font-size:11.9998827px;line-height:0.01%;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';font-variant-ligatures:normal;font-variant-caps:normal;font-variant-numeric:normal;font-feature-settings:normal;text-align:start;letter-spacing:0px;word-spacing:0px;writing-mode:lr-tb;text-anchor:start;fill:#000000;fill-opacity:1;stroke:none;stroke-width:1px;stroke-linecap:butt;stroke-linejoin:miter;stroke-opacity:1"
|
||||
transform="matrix(0.34071173,0,0,1.1975799,525.65361,723.00863)"><flowRegion
|
||||
transform="matrix(0.34071173,0,0,1.1975799,310.0168,858.19092)"><flowRegion
|
||||
id="flowRegion11267"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;font-size:11.9998827px;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';font-variant-ligatures:normal;font-variant-caps:normal;font-variant-numeric:normal;font-feature-settings:normal;text-align:start;writing-mode:lr-tb;text-anchor:start"><rect
|
||||
id="rect11269"
|
||||
@ -1932,7 +1932,7 @@
|
||||
y="252.68971"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;font-size:11.9998827px;line-height:125%;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';font-variant-ligatures:normal;font-variant-caps:normal;font-variant-numeric:normal;font-feature-settings:normal;text-align:start;writing-mode:lr-tb;text-anchor:start" /></flowRegion><flowPara
|
||||
id="flowPara3435">\shortstack{$\Lk(\alpha, \beta) = 3$}</flowPara></flowRoot> <flowRoot
|
||||
transform="matrix(0.39968512,0,0,0.29332933,182.9764,926.96522)"
|
||||
transform="matrix(0.18437441,0,0,0.1863568,280.05202,913.80836)"
|
||||
style="font-style:normal;font-variant:normal;font-weight:normal;font-stretch:normal;line-height:0.01%;font-family:sans-serif;-inkscape-font-specification:'sans-serif, Normal';text-align:start;letter-spacing:0px;word-spacing:0px;writing-mode:lr-tb;text-anchor:start;fill:#ff0000;fill-opacity:1;stroke:none;stroke-width:1px;stroke-linecap:butt;stroke-linejoin:miter;stroke-opacity:1"
|
||||
id="flowRoot11275"
|
||||
xml:space="preserve"><flowRegion
|
||||
|
||||
|
Before 
(image error) Size: 71 KiB After 
(image error) Size: 71 KiB 

|
@ -49,16 +49,16 @@
|
||||
\global\let\svgscale\undefined%
|
||||
\makeatother%
|
||||
\begin{picture}(1,0.36122373)%
|
||||
\put(0.76646668,0.87323963){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.30136242\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.76646668,0.87323963){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.30136242\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.58791127,0.62441177){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.06104295\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.63785551,0.61775254){\color[rgb]{0,0,0}\makebox(0,0)[lb]{\smash{ }}}%
|
||||
\put(1.53074757,-0.65083121){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.08878978\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(1.59789484,-0.63917748){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.0105438\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(1.57736214,-0.64139722){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.08989959\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.52853312,0.49733142){\color[rgb]{0,0,0}\makebox(0,0)[lb]{\smash{ }}}%
|
||||
\put(0.63785551,0.61775254){\color[rgb]{0,0,0}\makebox(0,0)[lb]{\smash{ }}}%
|
||||
\put(1.53074757,-0.65083121){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.08878978\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(1.59789484,-0.63917748){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.0105438\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(1.57736214,-0.64139722){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.08989959\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.52853312,0.49733142){\color[rgb]{0,0,0}\makebox(0,0)[lb]{\smash{ }}}%
|
||||
\put(0,0){\includegraphics[width=\unitlength,page=1]{seifert_matrix.pdf}}%
|
||||
\put(0.62239545,0.32756023){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.15393011\unitlength}\raggedright $\alpha_1^+$\end{minipage}}}%
|
||||
\put(0.69294518,0.43731309){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.14012473\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.69294518,0.43731309){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.14012473\unitlength}\raggedright \end{minipage}}}%
|
||||
\put(0.68037809,0.26129435){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.12493878\unitlength}\raggedright $\alpha_1$\end{minipage}}}%
|
||||
\put(0,0){\includegraphics[width=\unitlength,page=2]{seifert_matrix.pdf}}%
|
||||
\put(0.13859342,0.26031816){\color[rgb]{0,0,0}\makebox(0,0)[lt]{\begin{minipage}{0.12493878\unitlength}\raggedright $\alpha_1$\end{minipage}}}%
|
||||
|
||||
Binary file not shown.
|
Before 
(image error) Size: 13 KiB After 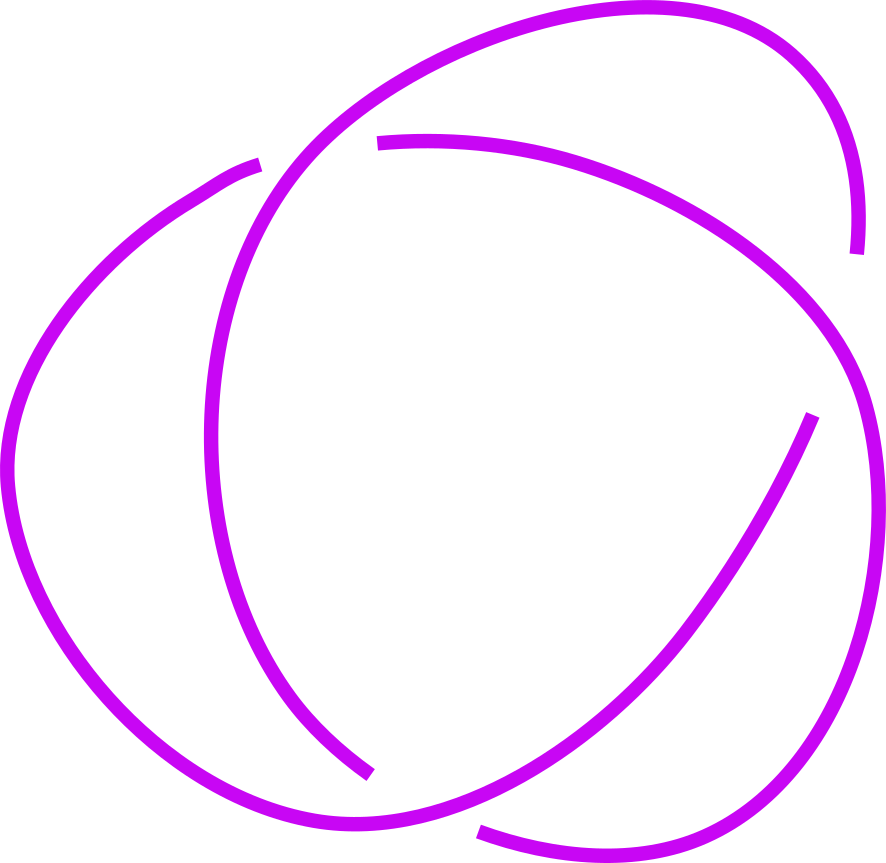
(image error) Size: 60 KiB 

|
1036
images/trefoil.svg
Normal file
1036
images/trefoil.svg
Normal file
File diff suppressed because it is too large
Load Diff
|
After 
(image error) Size: 36 KiB |
Binary file not shown.
|
Before 
(image error) Size: 4.2 KiB After 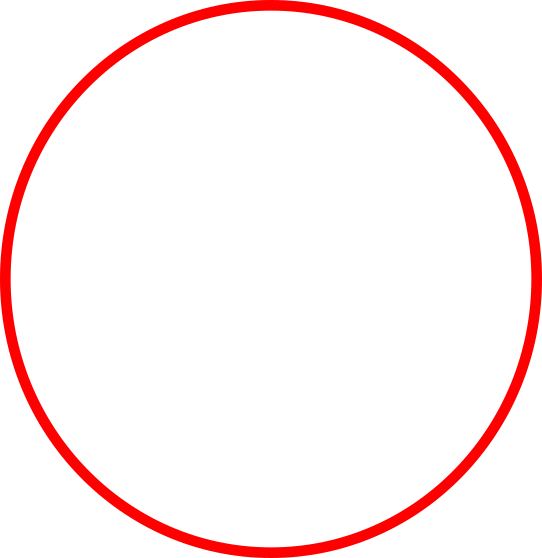
(image error) Size: 15 KiB 

|
119
images/unknot.svg
Normal file
119
images/unknot.svg
Normal file
@ -0,0 +1,119 @@
|
||||
<?xml version="1.0" encoding="UTF-8" standalone="no"?>
|
||||
<!-- Created with Inkscape (http://www.inkscape.org/) -->
|
||||
|
||||
<svg
|
||||
xmlns:dc="http://purl.org/dc/elements/1.1/"
|
||||
xmlns:cc="http://creativecommons.org/ns#"
|
||||
xmlns:rdf="http://www.w3.org/1999/02/22-rdf-syntax-ns#"
|
||||
xmlns:svg="http://www.w3.org/2000/svg"
|
||||
xmlns="http://www.w3.org/2000/svg"
|
||||
xmlns:sodipodi="http://sodipodi.sourceforge.net/DTD/sodipodi-0.dtd"
|
||||
xmlns:inkscape="http://www.inkscape.org/namespaces/inkscape"
|
||||
width="210mm"
|
||||
height="297mm"
|
||||
viewBox="0 0 210 297"
|
||||
version="1.1"
|
||||
id="svg8"
|
||||
inkscape:version="0.92.2 5c3e80d, 2017-08-06"
|
||||
sodipodi:docname="unknot.svg">
|
||||
<defs
|
||||
id="defs2">
|
||||
<inkscape:perspective
|
||||
sodipodi:type="inkscape:persp3d"
|
||||
inkscape:vp_x="0 : 148.5 : 1"
|
||||
inkscape:vp_y="0 : 1000 : 0"
|
||||
inkscape:vp_z="210 : 148.5 : 1"
|
||||
inkscape:persp3d-origin="105 : 99 : 1"
|
||||
id="perspective856" />
|
||||
<inkscape:path-effect
|
||||
effect="spiro"
|
||||
id="path-effect831"
|
||||
is_visible="true" />
|
||||
</defs>
|
||||
<sodipodi:namedview
|
||||
id="base"
|
||||
pagecolor="#ffffff"
|
||||
bordercolor="#666666"
|
||||
borderopacity="1.0"
|
||||
inkscape:pageopacity="0.0"
|
||||
inkscape:pageshadow="2"
|
||||
inkscape:zoom="0.57192761"
|
||||
inkscape:cx="55.898154"
|
||||
inkscape:cy="561.25984"
|
||||
inkscape:document-units="mm"
|
||||
inkscape:current-layer="layer1"
|
||||
showgrid="false"
|
||||
inkscape:window-width="1397"
|
||||
inkscape:window-height="855"
|
||||
inkscape:window-x="0"
|
||||
inkscape:window-y="1"
|
||||
inkscape:window-maximized="1" />
|
||||
<metadata
|
||||
id="metadata5">
|
||||
<rdf:RDF>
|
||||
<cc:Work
|
||||
rdf:about="">
|
||||
<dc:format>image/svg+xml</dc:format>
|
||||
<dc:type
|
||||
rdf:resource="http://purl.org/dc/dcmitype/StillImage" />
|
||||
<dc:title />
|
||||
</cc:Work>
|
||||
</rdf:RDF>
|
||||
</metadata>
|
||||
<g
|
||||
inkscape:label="Layer 1"
|
||||
inkscape:groupmode="layer"
|
||||
id="layer1">
|
||||
<ellipse
|
||||
style="opacity:0;fill:#ff0000;fill-opacity:1;fill-rule:evenodd;stroke:#a87873;stroke-width:7.76499987;stroke-linejoin:bevel;stroke-miterlimit:4;stroke-dasharray:none;stroke-dashoffset:0;stroke-opacity:1"
|
||||
id="path819"
|
||||
cx="109.23512"
|
||||
cy="132.58035"
|
||||
rx="72.949409"
|
||||
ry="57.074402" />
|
||||
<ellipse
|
||||
style="opacity:0;fill:#ff0000;fill-opacity:1;fill-rule:evenodd;stroke:#a87873;stroke-width:7.76499987;stroke-linejoin:bevel;stroke-miterlimit:4;stroke-dasharray:none;stroke-dashoffset:0;stroke-opacity:1"
|
||||
id="path821"
|
||||
cx="60.476189"
|
||||
cy="36.952377"
|
||||
rx="7.5595236"
|
||||
ry="13.607142" />
|
||||
<ellipse
|
||||
style="opacity:0;fill:#ff0000;fill-opacity:1;fill-rule:evenodd;stroke:#a87873;stroke-width:7.76499987;stroke-linejoin:bevel;stroke-miterlimit:4;stroke-dasharray:none;stroke-dashoffset:0;stroke-opacity:1"
|
||||
id="path823"
|
||||
cx="63.147194"
|
||||
cy="125.83177"
|
||||
rx="42.792053"
|
||||
ry="38.859814" />
|
||||
<ellipse
|
||||
style="opacity:0;fill:#550000;fill-opacity:1;fill-rule:evenodd;stroke:#a87873;stroke-width:7.76499987;stroke-linejoin:bevel;stroke-miterlimit:4;stroke-dasharray:none;stroke-dashoffset:0;stroke-opacity:1"
|
||||
id="path825"
|
||||
cx="118.42991"
|
||||
cy="98.999992"
|
||||
rx="37.934578"
|
||||
ry="35.621494" />
|
||||
<ellipse
|
||||
style="opacity:0;fill:#550000;fill-opacity:1;fill-rule:evenodd;stroke:#a87873;stroke-width:7.76499987;stroke-linejoin:bevel;stroke-miterlimit:4;stroke-dasharray:none;stroke-dashoffset:0;stroke-opacity:1"
|
||||
id="path827"
|
||||
cx="102.007"
|
||||
cy="171.39954"
|
||||
rx="14.109813"
|
||||
ry="39.553738" />
|
||||
<ellipse
|
||||
style="opacity:0;fill:#550000;fill-opacity:1;fill-rule:evenodd;stroke:#a87873;stroke-width:7.76499987;stroke-linejoin:bevel;stroke-miterlimit:4;stroke-dasharray:none;stroke-dashoffset:0;stroke-opacity:1"
|
||||
id="path833"
|
||||
cx="95.299065"
|
||||
cy="208.40887"
|
||||
rx="46.261681"
|
||||
ry="36.778034" />
|
||||
<ellipse
|
||||
style="opacity:1;fill:none;fill-opacity:1;fill-rule:evenodd;stroke:#ff0000;stroke-width:3;stroke-linejoin:bevel;stroke-miterlimit:4;stroke-dasharray:none;stroke-dashoffset:0;stroke-opacity:1"
|
||||
id="path845"
|
||||
cx="100.38785"
|
||||
cy="147.11214"
|
||||
rx="74.943924"
|
||||
ry="77.257011"
|
||||
inkscape:export-xdpi="90"
|
||||
inkscape:export-ydpi="90" />
|
||||
</g>
|
||||
</svg>
|
||||
|
After 
(image error) Size: 4.2 KiB |
@ -2,7 +2,7 @@
|
||||
%\begin{theorem}
|
||||
%For any knot $K \subset S^3$ there exists a connected, compact and orientable surface $\Sigma(K)$ such that $\partial \Sigma(K) = K$
|
||||
%\end{theorem}
|
||||
\begin{proof}(Theorem \ref{theo:Seifert})\\
|
||||
\begin{proof}(\Cref{theo:Seifert})\\
|
||||
Let $K \in S^3$ be a knot and $N = \nu(K)$ be its tubular neighbourhood. Because $K$ and $N$ are homotopy equivalent, we get:
|
||||
\begin{align*}
|
||||
H^1(S^3 \setminus N ) \cong H^1(S^3 \setminus K).
|
||||
|
||||
495
lec_25_02.tex
495
lec_25_02.tex
@ -1,69 +1,104 @@
|
||||
\begin{definition}
|
||||
A knot $K$ in $S^3$ is a smooth (PL - smooth) embedding of a circle $S^1$ in $S^3$:
|
||||
\begin{align*}
|
||||
\varphi: S^1 \hookrightarrow S^3
|
||||
\end{align*}
|
||||
A knot $K$ in $S^3$ is a smooth (PL - smooth)
|
||||
embedding of a circle $S^1$ in $S^3$:
|
||||
\[
|
||||
\varphi: S^1 \hookrightarrow S^3
|
||||
\]
|
||||
\end{definition}
|
||||
|
||||
\noindent
|
||||
Usually we think about a knot as an image of an embedding: $K = \varphi(S^1)$.
|
||||
Some basic examples and counterexamples are shown respectively in \autoref{fig:unknot} and \autoref{fig:notknot}.
|
||||
\begin{example}
|
||||
Usually we think about a knot
|
||||
as an image of an embedding:
|
||||
$K = \varphi(S^1)$.
|
||||
Some basic examples and counterexamples
|
||||
are shown respectively in
|
||||
\autoref{fig:unknot} and
|
||||
\autoref{fig:notknot}.
|
||||
|
||||
\begin{figure}[h]
|
||||
\includegraphics[width=0.08\textwidth]
|
||||
{unknot.png}
|
||||
\caption{Knots examples: unknot (left) and trefoil (right).}
|
||||
\label{fig:unknot}
|
||||
\centering
|
||||
\begin{subfigure}{0.3\textwidth}
|
||||
\centering
|
||||
\includegraphics[width=0.5\textwidth]
|
||||
{unknot.png}
|
||||
\end{subfigure}
|
||||
\begin{subfigure}{0.3\textwidth}
|
||||
\centering
|
||||
\includegraphics[width=0.5\textwidth]
|
||||
{trefoil.png}
|
||||
\end{subfigure}
|
||||
\caption{Knots examples:
|
||||
unknot (left) and trefoil (right).}
|
||||
\label{fig:unknot}
|
||||
\end{figure}
|
||||
|
||||
\begin{figure}[h]
|
||||
\includegraphics[width=0.08\textwidth]
|
||||
{unknot.png}
|
||||
\caption{Knots examples: unknot (left) and trefoil (right).}
|
||||
\label{fig:notknot}
|
||||
\centering
|
||||
\begin{subfigure}{0.3\textwidth}
|
||||
\centering
|
||||
\includegraphics[width=0.5\textwidth]
|
||||
{not_injective_knot.png}
|
||||
\end{subfigure}
|
||||
\begin{subfigure}{0.3\textwidth}
|
||||
\centering
|
||||
\includegraphics[width=0.5\textwidth]
|
||||
{not_smooth_knot.png}
|
||||
\end{subfigure}
|
||||
\caption{Not-knots examples:
|
||||
an image of
|
||||
a function ${S^1\longrightarrow S^3}$
|
||||
that isn't injective (left) and
|
||||
of a function
|
||||
that isn't smooth (right).}
|
||||
\label{fig:notknot}
|
||||
\end{figure}
|
||||
|
||||
|
||||
|
||||
\begin{itemize}
|
||||
\item
|
||||
Knots:
|
||||
\includegraphics[width=0.08\textwidth]{unknot.png} (unknot),
|
||||
\includegraphics[width=0.08\textwidth]{trefoil.png} (trefoil).
|
||||
\item
|
||||
Not knots:
|
||||
\includegraphics[width=0.12\textwidth]{not_injective_knot.png}
|
||||
(it is not an injection),
|
||||
\includegraphics[width=0.08\textwidth]{not_smooth_knot.png}
|
||||
(it is not smooth).
|
||||
\end{itemize}
|
||||
\end{example}
|
||||
\begin{definition}
|
||||
%\hfill\\
|
||||
Two knots $K_0 = \varphi_0(S^1)$, $K_1 = \varphi_1(S^1)$ are equivalent if the embeddings $\varphi_0$ and $\varphi_1$ are isotopic, that is there exists a continues function
|
||||
\begin{align*}
|
||||
&\Phi: S^1 \times [0, 1] \hookrightarrow S^3, \\
|
||||
&\Phi(x, t) = \Phi_t(x)
|
||||
\end{align*}
|
||||
such that $\Phi_t$ is an embedding for any $t \in [0,1]$, $\Phi_0 = \varphi_0$ and
|
||||
$\Phi_1 = \varphi_1$.
|
||||
Two knots $K_0 = \varphi_0(S^1)$,
|
||||
$K_1 = \varphi_1(S^1)$
|
||||
are equivalent if the embeddings
|
||||
$\varphi_0$ and $\varphi_1$ are isotopic,
|
||||
that is there exists a continues function
|
||||
\begin{align*}
|
||||
&\Phi: S^1 \times
|
||||
[0, 1] \hookrightarrow S^3, \\
|
||||
&\Phi(x, t) = \Phi_t(x)
|
||||
\end{align*}
|
||||
such that
|
||||
$\Phi_t$ is an embedding
|
||||
for any $t \in [0,1]$,
|
||||
$\Phi_0 = \varphi_0$ and
|
||||
$\Phi_1 = \varphi_1$.
|
||||
\end{definition}
|
||||
|
||||
\begin{theorem}
|
||||
Two knots $K_0$ and $K_1$ are isotopic if and only if they are ambient isotopic, i.e. there exists a family of self-diffeomorphisms $\Psi = \{\psi_t: t \in [0, 1]\}$ such that:
|
||||
\begin{align*}
|
||||
&\psi(t) = \psi_t \text{ is continius on $t\in [0,1]$},\\
|
||||
&\psi_t: S^3 \hookrightarrow S^3,\\
|
||||
& \psi_0 = id ,\\
|
||||
& \psi_1(K_0) = K_1.
|
||||
\end{align*}
|
||||
Two knots $K_0$ and $K_1$ are isotopic
|
||||
if and only if they are ambient isotopic,
|
||||
i.e. there exists a family of self-diffeomorphisms
|
||||
$\Psi = \{\psi_t: t \in [0, 1]\}$ such that:
|
||||
\begin{align*}
|
||||
&\psi(t) = \psi_t
|
||||
\text{ is continius on
|
||||
$t\in [0,1]$},\\
|
||||
&\psi_t: S^3 \hookrightarrow S^3,\\
|
||||
& \psi_0 = id ,\\
|
||||
& \psi_1(K_0) = K_1.
|
||||
\end{align*}
|
||||
\end{theorem}
|
||||
|
||||
\begin{definition}
|
||||
A knot is trivial (unknot) if it is equivalent to an embedding $\varphi(t) = (\cos t, \sin t, 0)$, where $t \in [0, 2 \pi] $ is a parametrisation of $S^1$.
|
||||
A knot is trivial (unknot) if it is equivalent
|
||||
to an embedding
|
||||
$\varphi(t) = (\cos t, \sin t, 0)$,
|
||||
where $t \in [0, 2 \pi] $
|
||||
is a parametrisation of $S^1$.
|
||||
\end{definition}
|
||||
|
||||
\begin{definition}
|
||||
A link with k - components is a (smooth) embedding of $\overbrace{S^1 \sqcup \ldots \sqcup S^1}^k$ in $S^3$.
|
||||
A link with $k$ - components is a
|
||||
(smooth) embedding of
|
||||
$\overbrace{S^1 \sqcup \ldots \sqcup S^1}^k$
|
||||
in $S^3$.
|
||||
\end{definition}
|
||||
|
||||
\begin{example}
|
||||
@ -85,20 +120,31 @@ a Borromean link:
|
||||
%
|
||||
%
|
||||
%
|
||||
\begin{definition}
|
||||
A link diagram $D_{\pi}$ is a picture over projection $\pi$ of a link $L$ in $\mathbb{R}^3$($S^3$) to $\mathbb{R}^2$ ($S^2$) such that:
|
||||
\begin{enumerate}[label={(\arabic*)}]
|
||||
\item
|
||||
$D_{\pi |_L}$ is non degenerate: \includegraphics[width=0.05\textwidth]{LinkDiagram1.png},
|
||||
\item the double points are not degenerate: \includegraphics[width=0.03\textwidth]{LinkDiagram2.png},
|
||||
\item there are no triple point: \includegraphics[width=0.05\textwidth]{LinkDiagram3.png}.
|
||||
\end{enumerate}
|
||||
\begin{definition}\label{def:link_diagram}
|
||||
A link diagram $D_{\pi}$ is a picture
|
||||
over projection $\pi$ of a link $L$ in
|
||||
$\mathbb{R}^3$($S^3$) to
|
||||
$\mathbb{R}^2$ ($S^2$) such that:
|
||||
\begin{enumerate}[label={(\arabic*)}]
|
||||
\item
|
||||
$D_{\pi |_L}$ is non degenerate,
|
||||
\item
|
||||
the double points are not degenerate,
|
||||
\item there are no triple point.
|
||||
\end{enumerate}
|
||||
\end{definition}
|
||||
\noindent
|
||||
By \Cref{def:link_diagram} the following pictures can not be a part of a diagram:
|
||||
\includegraphics[width=0.05\textwidth]{LinkDiagram1.png},
|
||||
\includegraphics[width=0.03\textwidth]{LinkDiagram2.png},
|
||||
\includegraphics[width=0.05\textwidth]{LinkDiagram3.png}.
|
||||
|
||||
|
||||
\noindent
|
||||
There are under- and overcrossings (tunnels and bridges) on a link diagrams with an obvious meaning.
|
||||
\begin{fact}
|
||||
\begin{lemma}
|
||||
Every link admits a link diagram.
|
||||
\end{fact}
|
||||
\end{lemma}
|
||||
\noindent
|
||||
|
||||
Let $D$ be a diagram of an oriented link (to each component of a link we add an arrow in the diagram).
|
||||
@ -108,15 +154,17 @@ $\left(\PICorientpluscross\right)$, called a positive crossing, and left-handed
|
||||
\subsection{Reidemeister moves}
|
||||
A Reidemeister move is one of the three types of operation on a link diagram as shown below:
|
||||
\begin{enumerate}[label=\Roman*]
|
||||
\item\hfill\\
|
||||
\includegraphics[width=0.6\textwidth]{rm1.png},
|
||||
\item\hfill\\\includegraphics[width=0.6\textwidth]{rm2.png},
|
||||
\item\hfill\\\includegraphics[width=0.4\textwidth]{rm3.png}.
|
||||
\item\hfill\\
|
||||
\includegraphics[width=0.6\textwidth]{rm1.png},
|
||||
\item\hfill\\\includegraphics[width=0.6\textwidth]{rm2.png},
|
||||
\item\hfill\\\includegraphics[width=0.4\textwidth]{rm3.png}.
|
||||
\end{enumerate}
|
||||
|
||||
\begin{theorem} [Reidemeister, 1927 ]
|
||||
Two diagrams of the same link can be
|
||||
deformed into each other by a finite sequence of Reidemeister moves (and isotopy of the plane).
|
||||
Two diagrams of the same link can
|
||||
be deformed into each other by a finite
|
||||
sequence of Reidemeister moves
|
||||
(and isotopy of the plane).
|
||||
\end{theorem}
|
||||
%
|
||||
%
|
||||
@ -134,169 +182,244 @@ deformed into each other by a finite sequence of Reidemeister moves (and isotopy
|
||||
\noindent
|
||||
Let $D$ be an oriented diagram of a link $L$. We change the diagram by smoothing each crossing:
|
||||
\begin{align*}
|
||||
\PICorientpluscross \mapsto \PICorientLRsplit,\\
|
||||
\PICorientminuscross \mapsto \PICorientLRsplit.
|
||||
\PICorientpluscross \mapsto
|
||||
\PICorientLRsplit,\\
|
||||
\PICorientminuscross \mapsto
|
||||
\PICorientLRsplit.
|
||||
\end{align*}
|
||||
We smooth all the crossings, so we get a disjoint union of circles on the plane. Each circle bounds a disks in $\mathbb{R}^3$ (we choose disks that don't intersect). For each smoothed crossing we add a twisted band: right-handed for a positive and left-handed for a negative one. We get an orientable surface $\Sigma$ such that $\partial \Sigma = L$.\\
|
||||
We smooth all the crossings, so we get
|
||||
a disjoint union of circles on the plane.
|
||||
Each circle bounds a disks in
|
||||
$\mathbb{R}^3$
|
||||
(we choose disks that don't intersect).
|
||||
For each smoothed crossing we add a twisted band: right-handed for a positive and left-handed for a negative one.
|
||||
We get an orientable surface $\Sigma$
|
||||
such that $\partial \Sigma = L$.\\
|
||||
|
||||
\begin{figure}[h]
|
||||
\fontsize{15}{10}\selectfont
|
||||
\centering{
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{0.8\textwidth}{!}{\input{images/seifert_alg.pdf_tex}}
|
||||
\caption{Constructing a Seifert surface.}
|
||||
\label{fig:SeifertAlg}
|
||||
}
|
||||
\fontsize{15}{10}\selectfont
|
||||
\centering{
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{0.8\textwidth}{!}
|
||||
{\input{images/seifert_alg.pdf_tex}}
|
||||
\caption{Constructing a Seifert surface.}
|
||||
\label{fig:SeifertAlg}
|
||||
}
|
||||
\end{figure}
|
||||
|
||||
\noindent
|
||||
Note: the obtained surface isn't unique and in general doesn't need to be connected, but by taking connected sum of all components we can easily get a connected surface (i.e. we take two disconnected components and cut a disk in each of them: $D_1$ and $D_2$. Then we glue both components on the boundaries: $\partial D_1$ and $\partial D_2$.
|
||||
|
||||
\begin{figure}[h]
|
||||
\begin{center}
|
||||
\includegraphics[width=0.6\textwidth]{seifert_connect.png}
|
||||
\end{center}
|
||||
\caption{Connecting two surfaces.}
|
||||
\label{fig:SeifertConnect}
|
||||
\centering
|
||||
\includegraphics[width=0.6\textwidth]
|
||||
{seifert_connect.png}
|
||||
\caption{Connecting two surfaces.}
|
||||
\label{fig:SeifertConnect}
|
||||
\end{figure}
|
||||
|
||||
\begin{theorem}[Seifert]
|
||||
\label{theo:Seifert}
|
||||
Every link in $S^3$ bounds a surface $\Sigma$ that is compact, connected and orientable. Such a surface is called a Seifert surface.
|
||||
\begin{theorem}[Seifert]\label{theo:Seifert}
|
||||
Every link in $S^3$ bounds a surface
|
||||
$\Sigma$ that is compact, connected
|
||||
and orientable.
|
||||
Such a surface is called a Seifert surface.
|
||||
\end{theorem}
|
||||
%
|
||||
|
||||
\begin{figure}[h]
|
||||
\fontsize{12}{10}\selectfont
|
||||
\centering{
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{1\textwidth}{!}{\input{images/torus_1_2_3.pdf_tex}}
|
||||
\caption{Genus of an orientable surface.}
|
||||
\label{fig:genera}
|
||||
}
|
||||
\fontsize{12}{10}\selectfont
|
||||
\centering
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{1\textwidth}{!}{
|
||||
\input{images/torus_1_2_3.pdf_tex}}
|
||||
\caption{Genus of an orientable surface.}
|
||||
\label{fig:genera}
|
||||
\end{figure}
|
||||
%
|
||||
%
|
||||
\begin{definition}
|
||||
The three genus $g_3(K)$ ($g(K)$) of a knot $K$ is the minimal genus of a Seifert surface $\Sigma$ for $K$.
|
||||
The three genus $g_3(K)$ ($g(K)$)
|
||||
of a knot $K$ is the minimal genus
|
||||
of a Seifert surface $\Sigma$ for $K$.
|
||||
\end{definition}
|
||||
|
||||
\begin{corollary}
|
||||
A knot $K$ is trivial if and only $g_3(K) = 0$.
|
||||
A knot $K$ is trivial if and only
|
||||
$g_3(K) = 0$.
|
||||
\end{corollary}
|
||||
|
||||
\noindent
|
||||
Remark: there are knots that admit non isotopic Seifert surfaces of minimal genus (András Juhász, 2008).
|
||||
Remark: there are knots that admit non isotopic
|
||||
Seifert surfaces of minimal genus
|
||||
(András Juhász, 2008).
|
||||
|
||||
\begin{definition}
|
||||
Suppose $\alpha$ and $\beta$ are two simple closed curves in $\mathbb{R}^3$.
|
||||
On a diagram $L$ consider all crossings between $\alpha$ and $\beta$. Let $N_+$ be the number of positive crossings, $N_-$ - negative. Then the linking number: $\Lk(\alpha, \beta) = \frac{1}{2}(N_+ - N_-)$.
|
||||
\end{definition}
|
||||
\begin{definition}
|
||||
\label{def:lk_via_homo}
|
||||
Let $\alpha$ and $\beta$ be two disjoint simple cross curves in $S^3$.
|
||||
Let $\nu(\beta)$ be a tubular neighbourhood of $\beta$. The linking number can be interpreted via first homology group, where $\Lk(\alpha, \beta)$ is equal to evaluation of $\alpha$ as element of first homology group of the complement of $\beta$:
|
||||
\[
|
||||
\alpha \in H_1(S^3 \setminus \nu(\beta), \mathbb{Z}) \cong \mathbb{Z}.\]
|
||||
Suppose $\alpha$ and $\beta$ are two
|
||||
simple closed curves in $\mathbb{R}^3$.
|
||||
On a diagram $L$ consider all crossings
|
||||
between $\alpha$ and $\beta$.
|
||||
Let $N_+$ be the number
|
||||
of positive crossings,
|
||||
$N_-$ - negative.
|
||||
Then the linking number:
|
||||
$\Lk(\alpha, \beta) =
|
||||
\frac{1}{2}(N_+ - N_-)$.
|
||||
\end{definition}
|
||||
|
||||
\begin{definition}\label{def:lk_via_homo}
|
||||
Let $\alpha$ and $\beta$ be
|
||||
two disjoint simple closed curves in $S^3$.
|
||||
Let $\nu(\beta)$ be a tubular
|
||||
neighbourhood of $\beta$.
|
||||
The linking number can be interpreted
|
||||
via first homology group, where
|
||||
$\Lk(\alpha, \beta)$ is equal
|
||||
to evaluation of $\alpha$ as element
|
||||
of first homology group
|
||||
of the complement of $\beta$:
|
||||
\[
|
||||
\alpha \in H_1(S^3 \setminus
|
||||
\nu(\beta), \mathbb{Z})
|
||||
\cong \mathbb{Z}.
|
||||
\]
|
||||
\end{definition}
|
||||
|
||||
\begin{example}
|
||||
\begin{itemize}
|
||||
\item
|
||||
A Hopf link:
|
||||
\begin{figure}[h]
|
||||
\fontsize{20}{10}\selectfont
|
||||
\centering{
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{0.4\textwidth}{!}{\input{images/linking_hopf.pdf_tex}},
|
||||
}
|
||||
\fontsize{10}{8}\selectfont
|
||||
\centering
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{\textwidth}{!}{
|
||||
% \centering
|
||||
\begin{subfigure}{0.3\textwidth}
|
||||
\centering
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{1\textwidth}{!}{
|
||||
\input{images/linking_torus_6_2.pdf_tex}
|
||||
}
|
||||
\end{subfigure}
|
||||
\begin{subfigure}{0.3\textwidth}
|
||||
\centering
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{1\textwidth}{!}{
|
||||
\input{images/linking_hopf.pdf_tex}
|
||||
}
|
||||
\end{subfigure}
|
||||
}
|
||||
\vspace*{10mm}
|
||||
\caption{
|
||||
Linking number of a Hopf link (left)
|
||||
and a torus link $T(6, 2)$ (right).
|
||||
}
|
||||
\label{fig:unknot}
|
||||
\end{figure}
|
||||
\item
|
||||
$T(6, 2)$ link:
|
||||
\begin{figure}[h]
|
||||
\fontsize{20}{10}\selectfont
|
||||
\centering{
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{0.4\textwidth}{!}{\input{images/linking_torus_6_2.pdf_tex}}.
|
||||
}
|
||||
\end{figure}
|
||||
\end{itemize}
|
||||
\end{example}
|
||||
|
||||
\begin{fact}
|
||||
$
|
||||
g_3(\Sigma) = \frac{1}{2} b_1 (\Sigma) =
|
||||
\frac{1}{2} \dim_{\mathbb{R}}H_1(\Sigma, \mathbb{R}),
|
||||
$
|
||||
where $b_1$ is first Betti number of $\Sigma$.
|
||||
where $b_1$ is first Betti number of a surface $\Sigma$.
|
||||
\end{fact}
|
||||
|
||||
\subsection{Seifert matrix}
|
||||
Let $L$ be a link and $\Sigma$ be an oriented Seifert surface for $L$. Choose a basis for $H_1(\Sigma, \mathbb{Z})$ consisting of simple closed curves $\alpha_1, \dots, \alpha_n$.
|
||||
Let $\alpha_1^+, \dots \alpha_n^+$ be copies of $\alpha_i$ lifted up off the surface (push up along a vector field normal to $\Sigma$). Note that elements $\alpha_i$ are contained in the Seifert surface while all $\alpha_i^+$ don't intersect the surface.
|
||||
Let $\Lk(\alpha_i, \alpha_j^+) = \{a_{ij}\}$. Then the matrix $S = \{a_{ij}\}_{i, j =1}^n$ is called a Seifert matrix for $L$. Note that by choosing a different basis we get a different matrix.
|
||||
Let $L$ be a link and
|
||||
$\Sigma$ be an oriented
|
||||
Seifert surface for $L$.
|
||||
Choose a basis for
|
||||
$H_1(\Sigma, \mathbb{Z})$
|
||||
consisting of simple closed curves
|
||||
$\alpha_1, \dots, \alpha_n$.
|
||||
|
||||
\noindent
|
||||
Let $\alpha_1^+, \dots \alpha_n^+$
|
||||
be copies of $\alpha_i$
|
||||
lifted up off the surface
|
||||
(push up along a vector field
|
||||
normal to $\Sigma$).
|
||||
Note that elements $\alpha_i$ are
|
||||
contained in the Seifert surface while all
|
||||
$\alpha_i^+$ don't intersect the surface.
|
||||
|
||||
\noindent
|
||||
Let $\Lk(\alpha_i, \alpha_j^+) = \{a_{ij}\}$.
|
||||
Then the matrix $S = \{a_{ij}\}_{i, j =1}^n$
|
||||
is called a Seifert matrix for $L$.
|
||||
Note that by choosing a different basis
|
||||
we get a different matrix.
|
||||
|
||||
\begin{figure}[h]
|
||||
\fontsize{20}{10}\selectfont
|
||||
\centering{
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{0.8\textwidth}{!}{\input{images/seifert_matrix.pdf_tex}}
|
||||
}
|
||||
\fontsize{20}{10}\selectfont
|
||||
\centering
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{0.8\textwidth}{!}{
|
||||
\input{images/seifert_matrix.pdf_tex}
|
||||
}
|
||||
\caption{
|
||||
A basis $\alpha_1, \alpha_2$
|
||||
of the first homology
|
||||
group of a Seifert surface
|
||||
and a copy of
|
||||
element $\alpha_1$ pushed up
|
||||
along vector normal to the Seifert surface.
|
||||
}
|
||||
\label{fig:alpha_plus}
|
||||
\end{figure}
|
||||
|
||||
\begin{theorem}
|
||||
The Seifert matrices $S_1$ and $S_2$ for the same link $L$ are S-equivalent, that is, $S_2$ can be obtained from $S_1$ by a sequence of following moves:
|
||||
\begin{enumerate}[label={(\arabic*)}]
|
||||
|
||||
\item
|
||||
$V \rightarrow AVA^T$, where $A$ is a matrix with integer coefficients,
|
||||
|
||||
\item
|
||||
|
||||
$V \rightarrow
|
||||
\begin{pmatrix}
|
||||
\begin{array}{c|c}
|
||||
V &
|
||||
\begin{matrix}
|
||||
\ast & 0 \\
|
||||
\sdots & \sdots\\
|
||||
\ast & 0
|
||||
\end{matrix} \\
|
||||
\hline
|
||||
\begin{matrix}
|
||||
\ast & \dots & \ast\\
|
||||
0 & \dots & 0
|
||||
\end{matrix}
|
||||
&
|
||||
\begin{matrix}
|
||||
0 & 0\\
|
||||
1 & 0
|
||||
\end{matrix}
|
||||
\end{array}
|
||||
\end{pmatrix} \quad$
|
||||
or
|
||||
$\quad
|
||||
V \rightarrow
|
||||
\begin{pmatrix}
|
||||
\begin{array}{c|c}
|
||||
V &
|
||||
\begin{matrix}
|
||||
\ast & 0 \\
|
||||
\sdots & \sdots\\
|
||||
\ast & 0
|
||||
\end{matrix} \\
|
||||
\hline
|
||||
\begin{matrix}
|
||||
\ast & \dots & \ast\\
|
||||
0 & \dots & 0
|
||||
\end{matrix}
|
||||
&
|
||||
\begin{matrix}
|
||||
0 & 1\\
|
||||
0 & 0
|
||||
\end{matrix}
|
||||
\end{array}
|
||||
\end{pmatrix},$
|
||||
\item
|
||||
inverse of (2).
|
||||
|
||||
\end{enumerate}
|
||||
The Seifert matrices $S_1$ and $S_2$
|
||||
for the same link $L$ are S-equivalent,
|
||||
that is, $S_2$ can be obtained from
|
||||
$S_1$ by a sequence of following moves:
|
||||
\begin{enumerate}[label={(\arabic*)}]
|
||||
\item
|
||||
$V \rightarrow AVA^T$,
|
||||
where $A$ is a matrix
|
||||
with integer coefficients,
|
||||
\item
|
||||
$V \rightarrow
|
||||
\begin{pmatrix}
|
||||
\begin{array}{c|c}
|
||||
V &
|
||||
\begin{matrix}
|
||||
\ast & 0 \\
|
||||
\sdots & \sdots\\
|
||||
\ast & 0
|
||||
\end{matrix} \\
|
||||
\hline
|
||||
\begin{matrix}
|
||||
\ast & \dots & \ast\\
|
||||
0 & \dots & 0
|
||||
\end{matrix}
|
||||
&
|
||||
\begin{matrix}
|
||||
0 & 0\\
|
||||
1 & 0
|
||||
\end{matrix}
|
||||
\end{array}
|
||||
\end{pmatrix} \quad$
|
||||
or
|
||||
$\quad
|
||||
V \rightarrow
|
||||
\begin{pmatrix}
|
||||
\begin{array}{c|c}
|
||||
V &
|
||||
\begin{matrix}
|
||||
\ast & 0 \\
|
||||
\sdots & \sdots\\
|
||||
\ast & 0
|
||||
\end{matrix} \\
|
||||
\hline
|
||||
\begin{matrix}
|
||||
\ast & \dots & \ast\\
|
||||
0 & \dots & 0
|
||||
\end{matrix}
|
||||
&
|
||||
\begin{matrix}
|
||||
0 & 1\\
|
||||
0 & 0
|
||||
\end{matrix}
|
||||
\end{array}
|
||||
\end{pmatrix},$
|
||||
\item
|
||||
inverse of (2).
|
||||
\end{enumerate}
|
||||
\end{theorem}
|
||||
|
||||
48
lec_mess.tex
Normal file
48
lec_mess.tex
Normal file
@ -0,0 +1,48 @@
|
||||
|
||||
|
||||
|
||||
|
||||
\begin{fact}[Milnor Singular Points of Complex Hypersurfaces]
|
||||
\end{fact}
|
||||
%\end{comment}
|
||||
\noindent
|
||||
An oriented knot is called negative amphichiral if the mirror image $m(K)$ of $K$ is equivalent the reverse knot of $K$: $K^r$. \\
|
||||
\begin{problem}
|
||||
Prove that if $K$ is negative amphichiral, then $K \# K = 0$ in
|
||||
$\mathscr{C}$.
|
||||
%
|
||||
%\\
|
||||
%Hint: $ -K = m(K)^r = (K^r)^r = K$
|
||||
\end{problem}
|
||||
\begin{example}
|
||||
Figure 8 knot is negative amphichiral.
|
||||
\end{example}
|
||||
%
|
||||
%
|
||||
\begin{theorem}
|
||||
Let $H_p$ be a $p$ - torsion part of $H$. There exists an orthogonal decomposition of $H_p$:
|
||||
\[
|
||||
H_p = H_{p, 1} \oplus \dots \oplus H_{p, r_p}.
|
||||
\]
|
||||
$H_{p, i}$ is a cyclic module:
|
||||
\[
|
||||
H_{p, i} = \quot{\mathbb{Z}[t, t^{-1}]}{p^{k_i} \mathbb{Z} [t, t^{-1}]}
|
||||
\]
|
||||
\end{theorem}
|
||||
\noindent
|
||||
The proof is the same as over $\mathbb{Z}$.
|
||||
\noindent
|
||||
%Add NotePrintSaveCiteYour opinionEmailShare
|
||||
%Saveliev, Nikolai
|
||||
|
||||
%Lectures on the Topology of 3-Manifolds
|
||||
%An Introduction to the Casson Invariant
|
||||
|
||||
\begin{figure}[h]
|
||||
\fontsize{10}{10}\selectfont
|
||||
\centering{
|
||||
\def\svgwidth{\linewidth}
|
||||
\resizebox{0.5\textwidth}{!}{\input{images/ball_4_alpha_beta.pdf_tex}}
|
||||
}
|
||||
%\caption{Sketch for Fact %%\label{fig:concordance_m}
|
||||
\end{figure}
|
||||
Binary file not shown.
@ -9,7 +9,9 @@
|
||||
|
||||
\usepackage[english]{babel}
|
||||
|
||||
\usepackage{caption}
|
||||
\usepackage[margin=1 cm]{caption}
|
||||
\usepackage{subcaption}
|
||||
%\usepackage{cleveref} - after hyperref
|
||||
\usepackage{comment}
|
||||
\usepackage{csquotes}
|
||||
|
||||
@ -21,6 +23,7 @@
|
||||
|
||||
\usepackage{graphicx}
|
||||
\usepackage{hyperref}
|
||||
\usepackage[nameinlink]{cleveref}
|
||||
|
||||
\usepackage{mathtools}
|
||||
|
||||
@ -28,6 +31,7 @@
|
||||
\usepackage[section]{placeins}
|
||||
\usepackage[pdf]{pstricks}
|
||||
|
||||
%\usepackage{subcaption} % added after caption
|
||||
\usepackage{tikz}
|
||||
\usepackage{titlesec}
|
||||
|
||||
@ -127,7 +131,7 @@
|
||||
|
||||
\begin{document}
|
||||
\tableofcontents
|
||||
%\newpage
|
||||
\newpage
|
||||
%\input{myNotes}
|
||||
|
||||
\section{Basic definitions
|
||||
@ -214,7 +218,7 @@ Surgery \hfill\DTMdate{2019-06-03}}
|
||||
\texorpdfstring{
|
||||
\hfill\DTMdate{2019-06-17}}
|
||||
{}}
|
||||
\input{mess.tex}
|
||||
\input{lec_mess.tex}
|
||||
|
||||
\end{document}
|
||||
|
||||
|
||||
Loading…
Reference in New Issue
Block a user